

Okay, hold on to your seats. We are about to take off into the world of properties of multiplication and it is sure to be a bumpy ride. Teaching students the properties of multiplication is a task, for sure, but it’s so important to build this foundation for kids.
Understanding the properties of multiplication is essential for unlocking the mysteries of numbers and operations. As educators, we strive to make complex mathematical concepts understandable and engaging for our students. But how can we effectively convey this to young learners?
If you want to build this foundation effectively, you’ll have to get creative. Hands-on activities that incorporate manipulatives and game-style practice are more likely to capture students’ attention and help them retain more of the information.
The goal is to teach properties of multiplication in a way that highlights its importance while providing ease of application. Let’s take a look at four multiplication properties and how you can apply this strategy with examples.
The commutative property of multiplication states that changing the order of the factors does not change the product. So, when multiplying two numbers, the product remains the same regardless of how you order the factors. Let’s explore some creative activities to teach the commutative property in an interactive and memorable way.
To introduce the commutative property of multiplication, we can begin with a simple explanation. We explain to students that no matter the order in which we multiply two numbers, the result, or product, remains the same. For instance, 2×3=3×2, and both equal 6.
To make this concept tangible, we can use dominos as a visual aid. We start by writing a multiplication equation on the board, such as 4×5. We then model this equation using dominos laid out in an array to represent the multiplication.
After demonstrating the array, we flip the domino and show that the factors are reversed, but the product remains the same.
After the initial demonstration, we encourage students to participate actively. We allow them to draw a domino from a pile and write a multiplication sentence based on the numbers they draw. Then, we provide objects for them to build an array in groups to find the product. Once they’ve constructed the array, we challenge them to flip the domino and write a second equation using the same factors.
To deepen understanding, we present students with a challenge. We ask them to change the array they’ve built without taking the entire thing apart. Through this exercise, students discover that they need the same number of pieces because the product remains constant, regardless of the arrangement of factors.
The distributive property of multiplication states that we can distribute or break down a multiplication problem into smaller, more manageable parts. It’s a fundamental principle that empowers students to break down multiplication problems into simpler equations.
For instance, 2×8 can be expressed as 2×4+2×4. By multiplying the parts separately and then adding the products together, we arrive at the same result.
To bring this concept to life, we kick off our lesson with a hands-on activity using playdough. We model a multiplication equation by building an array with playdough, representing the numbers involved. Then, we invite students to become mathematical ninjas as they “chop” the array into two smaller arrays.
As students split the array, we guide them in writing two separate equations for the smaller arrays created. For example, as seen above, if we start with an array representing 8 units and split it into arrays of 3 and 5 units, we demonstrate that 4×8 is equivalent to 4×3+4×5. Through this visual representation, students grasp the concept that breaking down one factor into smaller parts does not alter the overall product.
To solidify their understanding, we provide additional examples and encourage students to apply the distributive property on their own. By modeling and reinforcing the process of breaking down multiplication problems, we instill confidence and fluency in our students’ mathematical skills.
The associative property of multiplication is a cornerstone of mathematical reasoning that reveals the inherent symmetry and flexibility of mathematical operations.
The associative property of multiplication unveils a profound truth: the grouping of factors does not alter the product. Whether we multiply three or more numbers, the result remains constant regardless of how we group them. For instance, (3×4)×2=24 is equivalent to 3×(4×2)=24.
To illuminate this concept, we engage students in hands-on practice using number cards and manipulatives —whether M&Ms, counters, or blocks. The use of manipulatives is essential to making this abstract concept more tangible for kids and it adds a spark to a difficult topic.
They begin by building equations using their number cards, representing the factors involved in the multiplication problem.
Then, they will use their objects to demonstrate the associative property by strategically placing parentheses to group two of the numbers in different ways. They then use their objects to construct arrays that visually represent the multiplication problem.
By changing the grouping of numbers through the rearrangement of parentheses, students can easily observe that the product remains unchanged.
To reinforce their understanding, we provide additional examples and encourage students to explore different multiplication scenarios. Through guided practice and independent exploration, students internalize the concept of the associative property.
The multiplication property of zero states that any number multiplied by zero equals zero. The multiplication property of one, or the Identity Property, states that any number multiplied by one remains unchanged. In other words, its identity stays the same!
I’ll admit that this is one multiplication property that goes down a little easier for students, but it still deserves significant practice to ensure students understand how to apply it as more intricate concepts are introduced.
For example, in the multiplication sort above, students solve multiplication facts and sort based on this strategy.
You can grab this free activity plus additional multiplication printables below!
Teaching the properties of multiplication doesn’t have to be daunting. By incorporating hands-on activities and fun manipulatives like dominos and playdough, we can make abstract concepts more concrete and engaging for our students.
Interactive learning experiences allow students to deepen their understanding. So, let’s embrace creativity in our teaching!

Hey, y’all! My name is Amy Lemons and I am passionate about providing students with both engaging and effective standards-based Math and ELA lessons.
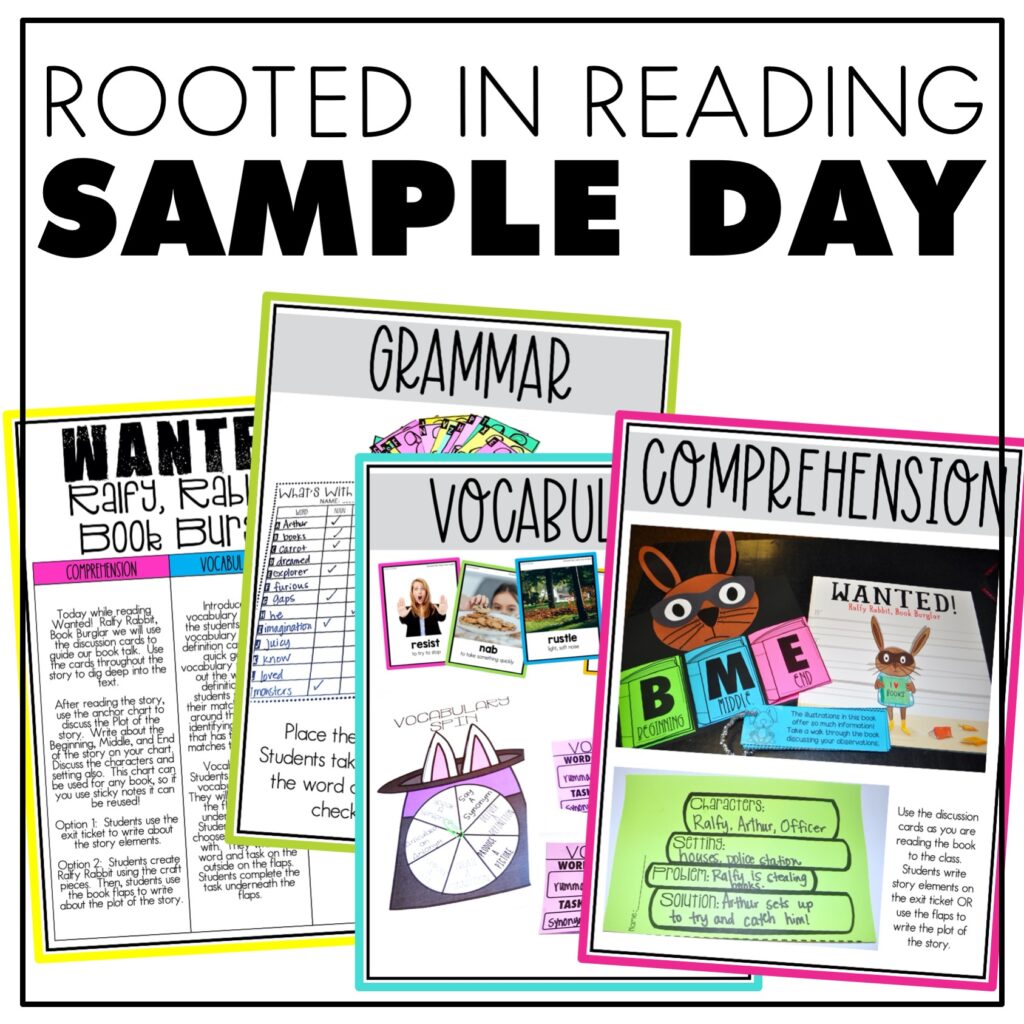
Sample a day of Rooted in Reading with these lesson plans and activities for Reading Comprehension, Vocabulary, and Grammar!